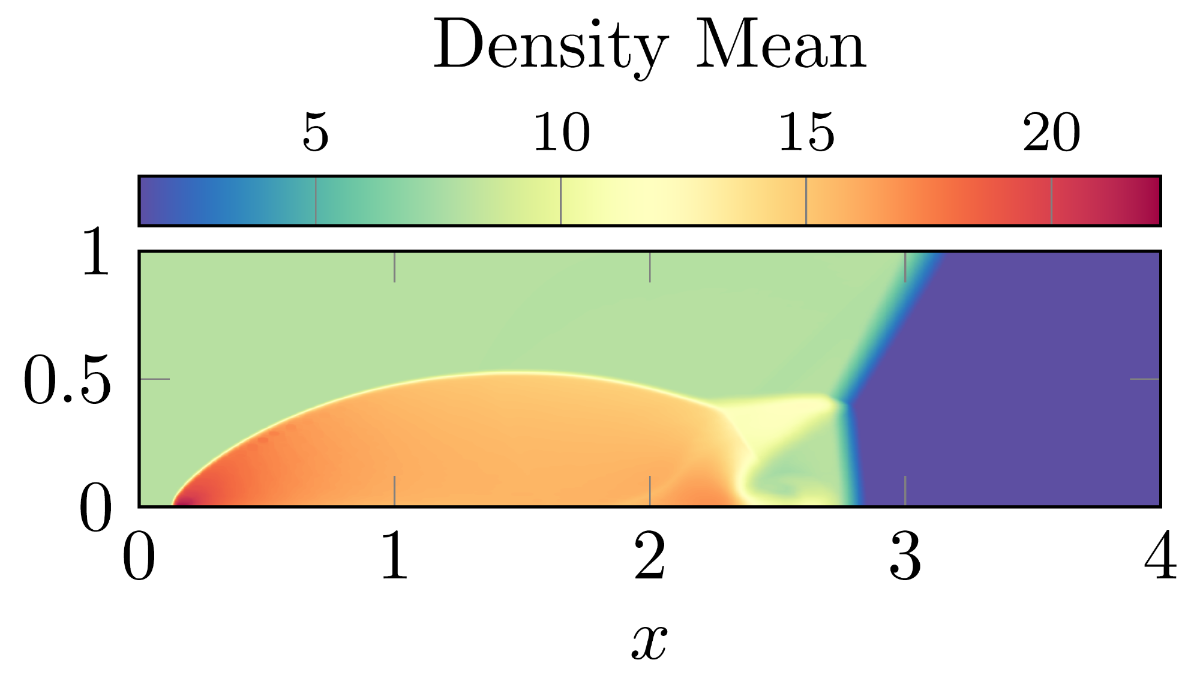
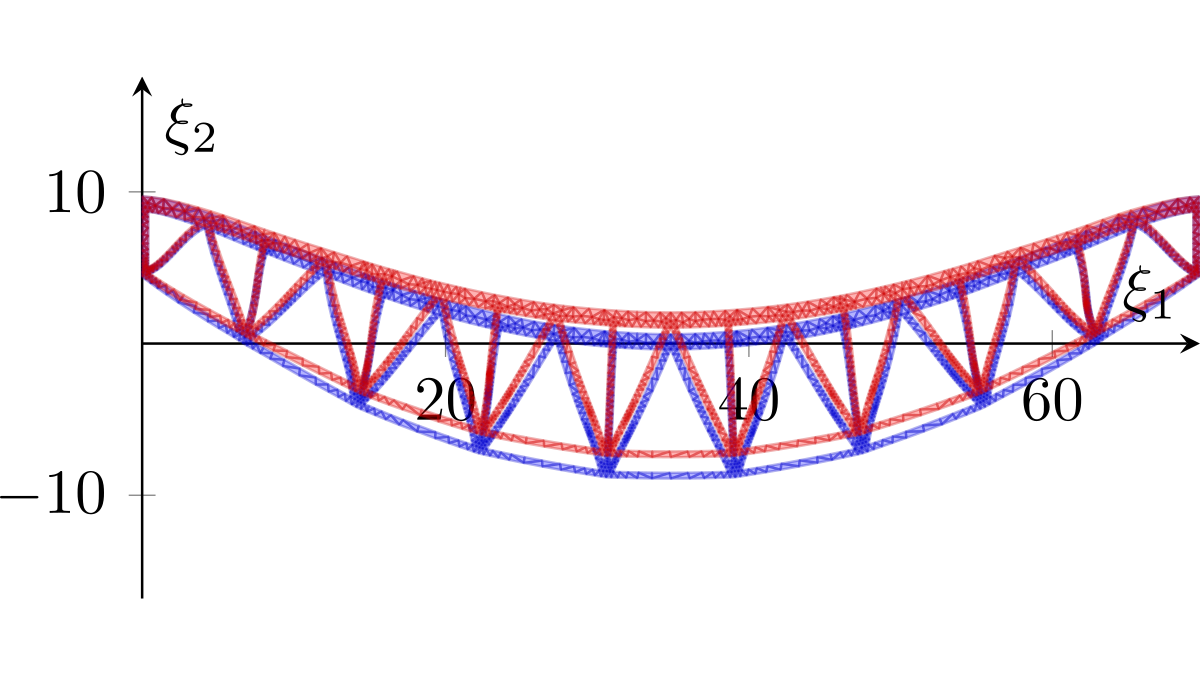
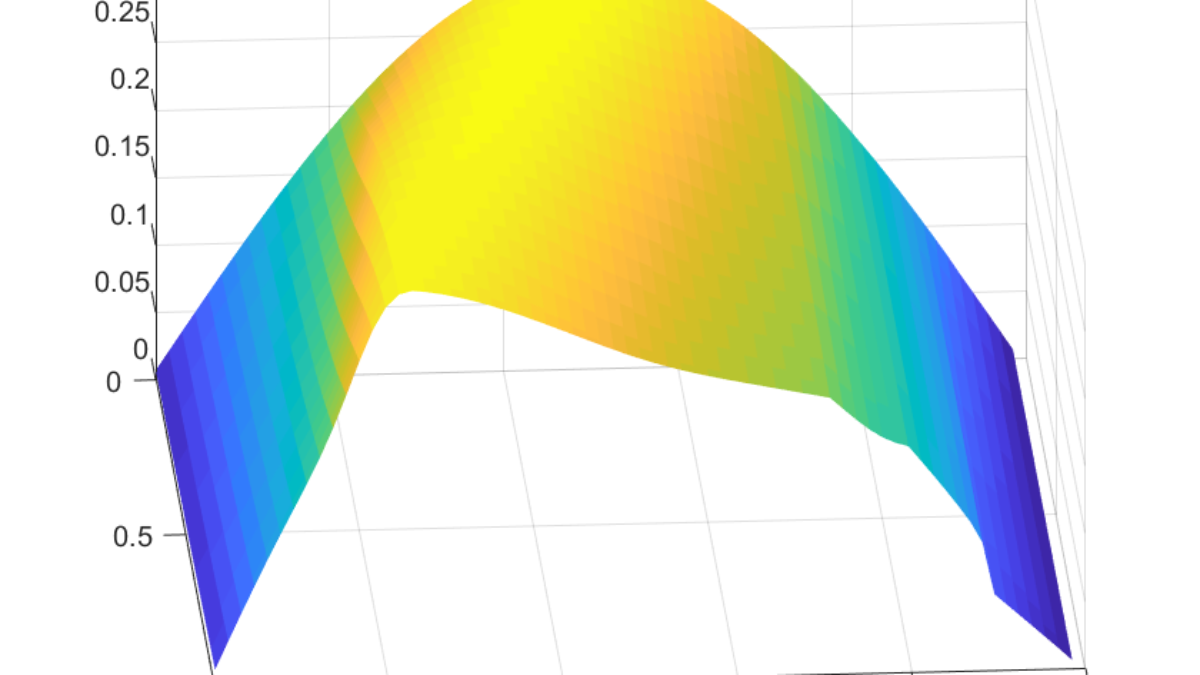
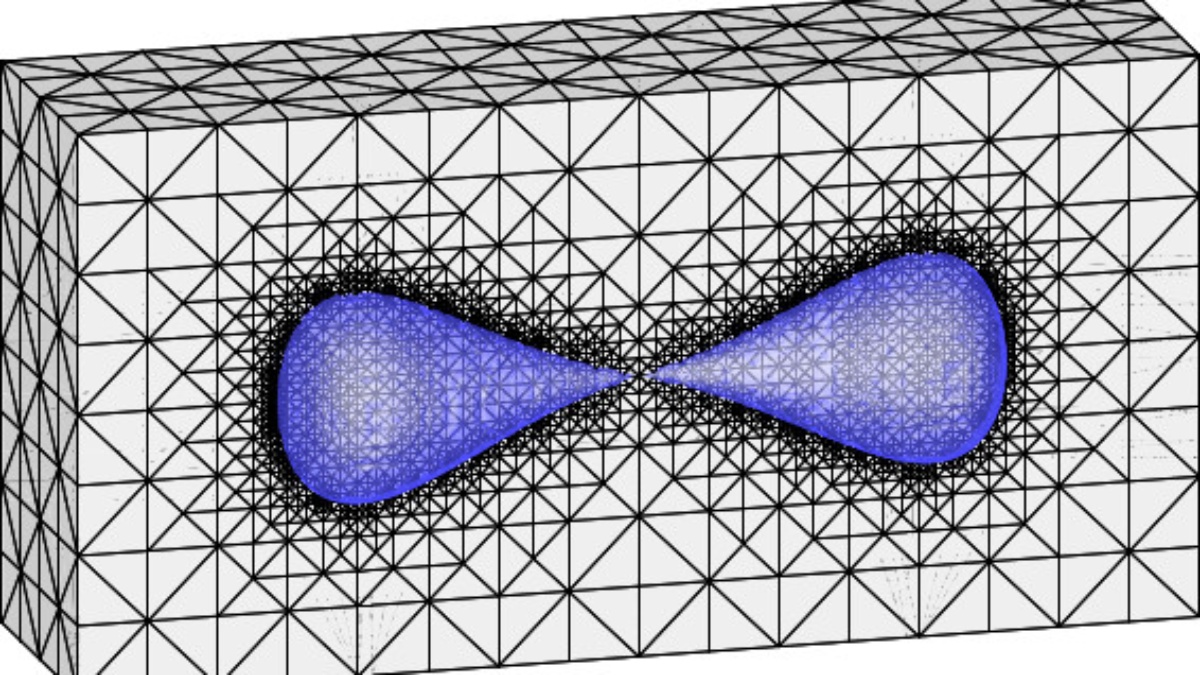
Quicklinks
Newsstream
-
Kundan Kumar received Mercator Fellowship Award
-
Congratulations to Maria Alkämper
Maria Alkämper successfully defended her Ph.D. thesis. -
Summer School on "Adaptive methods and a posteriori error estimation"
Hasselt University presents the Summer School from June 24-27, 2024 -
Prof. Dr. Anna-Margarete Sändig deceased
The Institute of Applied Analysis and Numerical Simulation mourns the death of Prof. Dr. Anna-Margarete Sändig. -
MOANSI 2023 in Stuttgart
Annual GAMM-specialist-committee-Meeting
Our research and teaching address a wide range of directions in modern applied mathematics: Mathematical modelling, applied analysis, stochastic techniques and scientific computing provide the theoretical and practical background to understand, predict and optimise highly complex processes in engineering, life, social and natural sciences. Numerical simulation has become the key technology to address these core questions of the University’s scientific and strategic orientation.
Our research involves developing, analyzing and implementing new numerical techniques in both problem-specific and more fundamental settings, and we are equally interested in multidisciplinary applications and theoretical foundations, connecting engineering, natural and computer sciences. We are involved in various national, international and industrial collaborations.
See below for more detailed information about our Institute.

Dominik Göddeke
Prof. Dr. rer. nat.Head of Institute and Head of Group

Britta Lenz
Secretary's Office